Introduction
From high school we know about the law of radioactive decay. According to this law, the number of undecayed atoms N at time T is related to the initial number of atoms N(T=0) by the formula
N(T)/N(0) = Exp(-T/T1) (1)
Т1 – is the average lifetime of an atom
From (1) it is not difficult to find the time when ½ of the undecayed atoms remains. From the equality Exp(-T/T1) =1/2 we find the half-life T=T0:
T0 = T1 · Ln2 ≈ T1· 0.7 (2)
Thus, the half-life is 30% shorter than the lifetime of an atom. It is important to note that after 2T0 all atoms will not decay, since each subsequent half-cycle T0 reduces the number of non-decayed (surviving) atoms by 2 times. Thus, the number of surviving atoms asymptotically tends to zero over time.
Half-life (T0) is a convenient physical constant (!) that characterizes the stability of a given type of nuclei (particles)
Brand retention (aging)
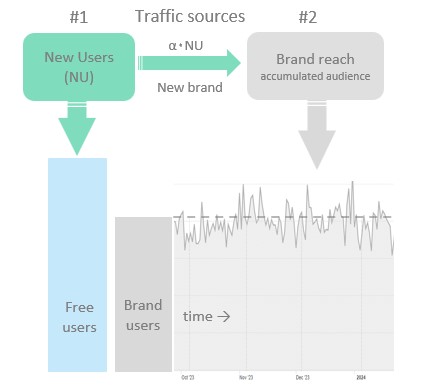
According to the definition, see help the Brandcounter, the traffic of any website (app) can be divided into Free and Brand. Figure 1 shows the sources of these 2 types of traffic.
Free users (FU) visit the site for only = 1 day and will never return to your resource again.
Brand users (BU) will visit your resource for 2 or more days (=2+ days) and the source of this traffic is the audience accumulated by your brand. Please note that part of the New Users (NU) is returned to the resource as a New brand (NB) and thereby makes up for the natural loss of the brand’s audience. The balance between the inflow of NB and the natural outflow of BU leads to time-stable traffic (see Fig. 1)
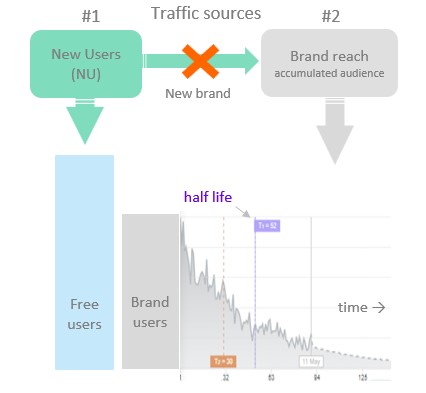
What happens if you cut off the source of replenishment of the audience accumulated by a brand? (see Fig. 2)
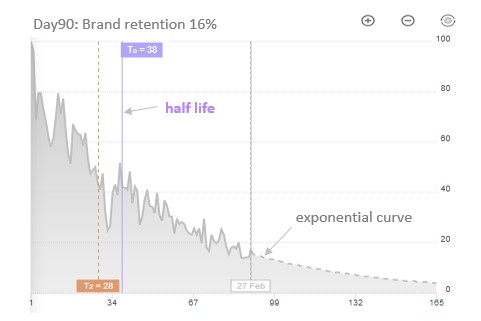
The brand’s audience will begin to age and die. Direct measurements show that the retention (aging) curve of a brand’s audience is well described by an exponential curve. Using the formalism of the law of radioactive decay, see formula (1) and (2), you can apply it to Brand.
The half-life of a brand, T0, is the period of time during which the accumulated audience of a brand will fall by 50%
It is important to note that the T0 score should be considered solely as a measure of a brand’s aging resistance. Obviously, for an ideal brand T0 →∞
If your brand traffic is approximately constant over time, then you can say that every period of time T0 your brand is updated by new brand users by 50%.
So far, no web analytics system measures the T0 brand half-life indicator and therefore there are no statistics for classifying brands as bad/good. Brandcounter is only at the beginning of its journey
Yuri Ryazanov
Feb. 2024

